[Series] Sum of Squares
?By: Tao Steven Zheng (鄭濤)
【Problem】
In his work "On Spirals", Archimedes (287 – 212 BC) derived the formula for calculating the sum of consecutive perfect squares. Figure 1 shows the geometric representation of the sum
used by Archimedes. He was able to derive the formula
Explain Archimedes’ proof of the sum of consecutive perfect squares using modern algebraic notation.
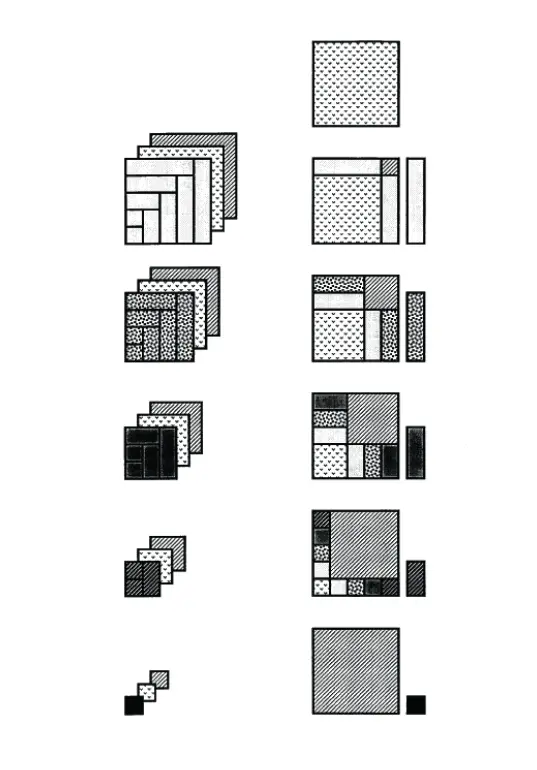

【Solution】
?Figure 1 represents the equation
Since
it follows that
Consequently,
標(biāo)簽:

